
Smith, R. D. (1998) 'Social
Structures and Chaos Theory'
Sociological Research Online,
vol. 3, no. 1, <http://www.socresonline.org.uk/3/1/11.html>
To cite articles published in Sociological Research Online, please reference the above information and include paragraph numbers if necessary
Received: 4/7/97 Accepted: 24/3/98 Published: 31/3/98
 Abstract
Abstract Structures
Structures
The term 'structure' may be defined as a pattern, ie. an observable uniformity, in terms of which action (or operations) takes place. ... Structure refers to an aspect of empirical phenomena that can be divorced from time. The patterns of action, qua patterns, do not exist as concrete objects in the same sense that sticks and stones do. The patterns of action in this sense are abstractions from concrete empirical phenomena, and they 'exist' and are empirically verifiable in the same sense that the squareness of a box exists and is empirically verifiable. (Levy, 1968)
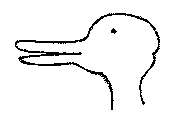
'I shall call this figure the duck-rabbit. It can be seen as a rabbit's head or as a duck's. And I must distinguish between the 'continuous seeing' of an aspect and the 'dawning' of an aspect. The picture might have been shown me, and I have never seen anything but a rabbit in it.' - Ludwig Wittgenstein (after Fuglesang, 1982).
Figure 1: Duck-Rabbit
| Subprocess or Condition | Types or Stages of Assimilation |
| Changing of cultural patterns to those of host society | Cultural or behavioural assimilation |
| Large-scale entrance into cliques, clubs, and institutions of host society, on primary group level | Structural assimilation |
| Large-scale intermarriage | Marital assimilation |
| Development of sense of people-hood based exclusively on host society | Identificational assimilation |
| Absence of prejudice | Attitude receptional assimilation |
| Absence of discrimination | Behaviour receptional assimilation |
| Absence of value and power conflict | Civic assimilation |
Figure 2: Ethnic Assimilation Variables (source: Gordon, 1964)
 Memes
Memes

Solitons are described mathematically as Kortweg-de Vries (or KdV) equations. They are of the form:
ut - 6uux - uxxx = 0
where: u (x,t) = exp{-k3t + ik(x-t)}; k 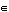 R, k
R, k 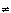 0
0
and: ux = 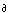 u/
u/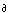 x
x
(from Drazin and Johnson, 1989)
The figure shows the computed dynamics of a two-soliton interaction. It is essential to realise this soliton is a wave travelling through a medium or some other matrix capable of transmission. Water waves, for example, pulse through the medium (oceans or lakes or rivers) but at every stage of their travel they are made up of different collections of water molecules. The water molecules which make up the wave at any given time are largely distinct. As complex as these waves are they are not infinitely complex (within a given dimensional space) unless the dimensions themselves are continuous. Water, coming in molecules, is quantised. The issue of infinitely variable waves must then rely to some extent on whether space (or space-time) itself is amenable to being infinitely subdivided or it is also quantised. (see for example: Misner et al (1973). This consideration will appear later when the specific case of the Lorenz attractor is examined.
Figure 3: Computer Rendering of a Two-Soliton Interaction
 Chaos
Chaos
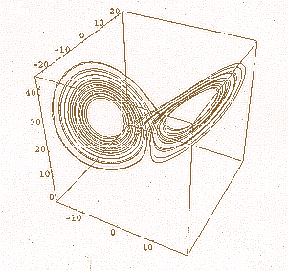
For true chaos to be present the orbital pathway must be a line of infinite length. If it passes through the same point twice then the deterministic nature of chaos informs us the orbit is of fixed periodicity and length. In order for true chaos to exist in nature, then, it may be necessary for space-time to be continuous and not quantised. If it is quantised then the number of possible positions is, while incomprehensibly large, finite. Conservation of energy means that the phase-space for such an orbit would likewise be limited. Lattice-models in which both chaos and solitons arise, however, have been proposed (Sayadi and Pouget, 1991).
Note that in this plot the lines clearly appear to intersect. They only appear to do so because this a two-dimensional rendering of a three-dimensional process. Chaos in a phase-space depends on the dimensions of the phase-space. If the actual dimensions of the connections (the lattice through which the signal or influence propagates) is of evolving or growing dimensionality then apparently finite restrictions may be ephemeral. See, however, for opposing views on themes related to this, Dyson (1988) and Gell-Mann (1994).
Figure 4: The Lorenz Attractor
 Complexity
Complexity
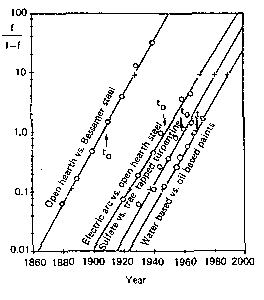
Market penetration of four processes or machines in the United States. The time constant is about 50 years for all four cases. Here the process is one way there are no cycles involved. The assumption is that the rate of these penetration processes is the primary clock of the economy. This rate may be closely linked to human behaviour and may be the deep reason for the stability of the cycles' length. (Marchetti, 1980)
Note that the power law here is based on a logistic relationship.
Marchetti's suspicion that a 'deep reason' exists for why these relationships all conform to a power-law relationship is arguably a manifestation of statistical self-similarity being detected by a researcher. Self-similarity results from two or more apparently distinct phenomena exhibiting commonalties. These commonalties apparently pique human curiosity and cause us to ask "is this merely a coincidence or is something else going on here?" Human psychology seems to be distrustful of assigning too many coincidences to the reason of random chance.
Self-similarity is also evident in the lattice approach of connectionism when people are in some ways networked together in patterns highly reminiscent of those which connect neurons in the brain. This is by no means either necessary or sufficient proof of the superiority of a connectionist paradigm but it is indeed adequate to justify further research and speculation in this area.
Figure 5: Power-Law Curves Exhibiting Market Penetration

A Trajectory
P' Æ P''
close to the degenerate heteroclinic loop.
where:
x(t)= the public support (popularity) of politicians at time t.
y(t) = the hidden assets that the corrupt politicians hold at time t.
z(t) = the investigation effort at time t (ie. the sum of activities by different
institutions such as police, courts, and the press).
and:
A = A(x); A representing positive actions taken
A*(x(t)); A* is the level of positive expectations held by the public
B = B(x,y); B representing bribes and all other moneys earned illegally by
politicians
C = C(B,y); C representing the private consumption of the politician as constrained by
bribes and hidden assets.
D = D(y,z); D representing the discovery of the corruption. (Here: the amount of money
confiscated by the courts).
And this leads to the following model:
Where ( represents the positive or negative reactions of the public, ( the persistence of investigations and ( represents the relative importance of manpower expended to dollars recovered.
Figure 6: Strange Attractor dynamics for the popularity of a corrupt politician (Rinaldi et al, 1994)
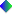 Connectionism
Connectionism
Two major connectivity dimensions are overlaid here. The 'mutual' and 'nonreciprocated' ties represent architectural features of this network. The flux patterns are a distinct type of structural category. The actual architecture only indicates what pathways the flux may follow and what qualitative kinds of ties they may be. Not all reasons for mutual choice are equal and so even though person 3 is mutually tied to both person 1 and person 2 this does not mean these two individuals have the same ability to influence person 3 to exploit any of his/her nonreciprocated ties. The idea of nonreciprocity is potentially subject to change as well. The architecture is thus not fixed permanently but only presumed to be constant for one or two cycles (iterations) of the flux structure. Excessive demands (or failures to engage in mutuality) could rupture some ties and create others. This network's architecture is itself capable of changing but it would likely do so at a slower rate than the actual transmission of information (or other communications) takes place.
The pioneering work of Hunter (1953) was one of the first systematic attempts to employ network concepts in the analysis of communities. Today's technology is still woefully inadequate to do carry out his project adequately.
Figure 7: Sociogram Showing Friendship Networks in a School Fraternity
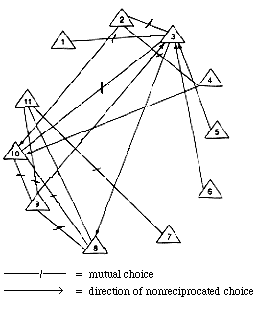
A network is saturated if all possible links are present. The density of the connections in (a) is almost saturated as only the A-D link is absent. In (b) the role of C is crucial and this status is sometimes called a 'sociometric star'. It could also be called a hub (as in hub-spoke airports) in airline industry terminology.
Figure 8: High and Low Density Networks
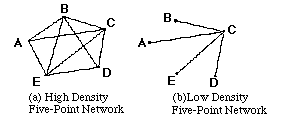
This network has three subgroups each of which is either connectively saturated or only one link short of saturation. Taken as a whole the network is moderately saturated. If one redefines a network as a group of connected elements with more than one pathway able to connect any two elements then this figure represents three networks. Using such a bright-line definition is essential for some kind of conceptual simplicity but as even this simple illustration reveals it is not totally accurate. In this rendering we assume Links A and B are in the same dimensions as the dimensions which connect the elements of X, Y, and Z.
Figure 9: Fuzzy Structure: A Network with Three Distinct Sub-groups
 Reductionism
Reductionism
 Transduction
Transduction
 Epistemology
Epistemology
 Summary
Summary
 Conclusions
Conclusions
![]()
 Notes
Notes2 Ethnicity is not the only area of sociological theorising which contains terminology which is, at best, overly general. Virtually everyone in society realises that what is called 'the family' has been in flux for several centuries. Attempts to articulate a formal sociological theory which logically (ie. in its 'formalism') allows change to be an integral component is seldom if ever seriously discussed.
3 meme: (pron. 'meem') A contagious idea that replicates like a virus, passed on from mind to mind. Memes function the same way genes and viruses do, propagating through communication networks and face-to-face contact between people. Root of the word 'memetics', a field of study which postulates that the meme is the basic unit of cultural evolution. Examples of memes range from melodies, icons, fashion statements and phrases to religious ideas, political ideologies, sexual mores, health beliefs and so on. The abstract entity manipulated by memory is known as a mnemon (Gabora, 1996).
4 The reliance of this on the 'subjective' or more accurately on the species-universal-but-relatively-subjective idea of what 'too rapidly' might mean is useful. It will allow us to begin formalising what is meant by the 'subjective/objective' distinction.
5 By 'easily' is meant 'intuitively obvious'. The actual mathematical models involved are extremely computation-intensive and computer technology well in advance of anything currently available would be minimally necessary to do any serious social modelling attempts.
6 Determining the dimensions for the soliton wave is crucial if this is to be more than just another metaphor misunderstood by and perhaps misleading to sociologists.
7 It is noted that almost all sociologists claim to believe this. The point is, though, that these phenomena must be accounted for by the formal logical relationships amongst the taxonomic entities of the paradigm under study (by 'the formalism' to adopt the usage from physics). In this terminology one might restate Homans' criticism as saying the formalism of structuralism has no real place for human consciousness.
8 There are at least two implications which result from this. With respect to classical 'structural-functional' sociological theory it means: the micro-macro distinction is without coherence. Within the realm of ethical theory it means any attempt to justify 'situation ethics' in terms of such arguments as 'the greatest good for the greatest number' (a form of consequentialist argument as discussed by Scheffler, 1982) is without any hope of being demonstrated empirically.
9 Pipes' theory is not alone in suggesting a so- called minor event leading to the Second World War and beyond. Calvin (1983) argues that President Woodrow Wilson's participation in the Paris peace conference of 1919 was drastically compromised by a bout of influenza. Prior to this incident his attitude towards Germany was to avoid humiliating it and indeed to include it as a member in the League of Nations. Following his illness he treated German delegates with contempt and was not a factor in preventing the imposition of the harsher features of the Treaty of Versailles.
Many feel that some of the consequences of Wilson's illness outlived his presidency ... and were to be seen in the events of the following decades - in the disastrous German inflation of the 1920s during the reparations exacted by the Allies, in the ensuing reaction to social disorder which led to the rise of the genocidal Nazis, and in the second world war. (Calvin, 1983: p. 124).
Juxtaposing the two thought experiments of Calvin and Pipes does two things: it illustrates how small changes in the initial conditions in an historical period might lead to radically different outcomes and, second, it draws us back to the question of system boundaries - of taxonomy - once again. For many reasons which are clearly legitimate one could in 1918 and again in 1998 regard Russia, Germany, and the USA as separate from each other. For other but equally legitimate reasons they must be seen as parts of larger entities. It is partly to deal with this exigency that 'connectionism' is introduced later in this paper. A final observation which can be made involves the realisation that despite the fact that historians often argue that 'laws of history' are demonstrably impossible as evidenced by the above complications, it is also the fact that some of their demonstrations do so by invidiously (in some cases) employing 'sociological laws' to reveal that more than one logically possible future scenario is possible. It is to be noted that the claim 'there are no laws of history' is epistemologically distinct from 'the laws of history do not yield a single and inescapable future scenario'. To discuss 'plausible futures' is to employ theory. For example: Had Wilson's health lasted a few more months and had Fannie Kaplan's memory been better how many of us alive today would ever have been born?
10 If the phase-space is sufficiently differentiated to allow for a single period longer than the effective of projected age of the universe then an interesting result is obtained. We may not be able to discriminate between a deterministic and a voluntaristic (free-will) condition for human existence.
11 There is a similar apparent irony in the doctrine of free will. Free Will is meaningless in a non-deterministic universe because it is the very determinism of the universe which permits on of the most common ways to exercise free will: that of selecting a course of action because of its consequences. The statement 'I choose to do X because doing X will cause Y to happen' contains both voluntaristic and deterministic elements.
12 The reductionism mandated by chaos theory derive from the requirement of a change or flux needing a matrix within which to manifest itself. The large-scale structures as studied by complexity theory are themselves rooted in the microstructure. One can at least speculate if the laws which may dictate the formation of 'social structures' are similar to 'brain structures'.
13 In more stilted language, the flux pattern is described in phase-space while the lattice exists in space alone. We leave to future time the discussion of the permanence of the lattice itself. People are just as transient as sticks and stones if divorces time from the system. (eg. Bohm and Hiley, 1993; Newton, 1993). For those who are unsure what is meant by 'phase spaces' and 'phase states' it is perhaps better to consult Gleick's (1988) extensive treatment of this idea. Schuster (1995) gives a more compressed discussion of the same ideas.
14 If the path is really finite then true chaos is impossible. If, however, the projected age of the universe is insufficiently great to allow even one iteration of the path then even though determinism was strictly present it might be impossible to detect this fact. This might, by extension, allow for the unassailable defence of a doctrine of free will, even if not one which is identical with the classical understanding.
15 The initial definition of structure makes the assertion that social structures do not exist in the same way concrete objects such as sticks and stones do. One is prompted to ask 'are human beings concrete objects or abstractions from concrete empirical phenomena'?
16 While Nagel's explanation of how the ideal gas law can be derived from the kinetic theory of gasses is logically satisfying it is less adequate as a sociology or psychological comment. Boyle's law relating temperature to pressure was postulated in 1662. Atoms had been proposed by Democritus (c460 B.C. - c370 B.C.) and some rudimentary concept of molecules also existed but at the same time both alchemy and astrology were considered scientific. The actual ideal gas law was generally accepted in the 1850s but this was almost one-half century after Avogadro published it in 1811. When Nagel wrote of the means by which the gas laws could be derived from the kinetic theory of gasses he lived in a world which accepted the existence of protons, neutrons and electrons (but not quarks).
17 Qualitative studies and their exponents (eg. Blumer) have often exhibited their greatest analytic strengths in documenting precisely these transitions. Those engaged in field research have long known of the limitations of formal structural theory.
18 All sciences must share the same methodological precepts even as they focus on different aspects of the natural world. Physics, biology, and chemistry therefore all share overarching notions of 'objectively collected data', of the formulation of testable hypotheses which are evaluated in accord to universal rules of logic and evidence, and the formulation of theories which generalise or extend observed patterns into areas which have not yet been observed. (This ability to extrapolate is intimately tied to the question of dimensions). 'Scientific method' at its core apparently means, reliance on logic, the use of empirical evidence, and the supremacy of evidence in case of conflict.
19 In a culturally more homogeneous age it made some statistical sense to refer to a 'family' as 'two adults of opposite sex, married to each other, and living with their common children'. While it may be politically advantageous (correct?) to extend the meaning of 'family' to include same-sex couples, single-parents, nannies, adoptive and step children, and so on, it is clear that this diversity plays havoc with sociological analysis. When a composite term is seen to be made up of elements which are varying essentially independently of one-another, scientific practice requires the composite term no longer be accorded primary theoretical, practical or research importance. After the tea kettle has boiled dry, using the term 'ice' or using predictions based on observations of ice are likely to be of diminished importance. The same can be argued about composite epistemological terms as well.
20 Positivism though, like liberalism, came to mean different things to different people. When Auguste Comte first proposed the Positive Philosophy he sought to study society with the tools of the natural sciences, forgoing both metaphysics and theology, in the quest for ways to make the lives of people better. By the time the critical theorists of the current period had arrived, positivism had come to be associated with the disenchantment of the world and the imposition of Western Rationalism on all and sundry. Positivism, like so many other sociological doctrines, is both a research methodology and a political promise. Many modern scientists attempt to avoid the Janus-headed positivism of Comte and restrict their interpretation to it's methodological role only: 'That philosophical attitude which asserts that science is solely concerned with directly measurable quantities and that its aim is simply the harmonious reconciliation of observations without attempting to speak of an underlying physical reality.' (Polkinghorne, 1986: p. 108)
21 No insult to either members of the Natural Law Party or Post-Modernists is intended here.
22 This assumption is more deceptive than it might first appear. The model proposed here fundamentally deals with an attempt to grapple with how things change once they have been set in motion. It does not deal with why or how they were set in motion in the first place. It is therefore not a model of cause and effect. It is only an attempt to model the flux of the real.
 Acknowledgements
Acknowledgements
BOHM, David and B.J. HILEY (1993) The Undivided Universe: An Ontological Interpretation of Quantum Theory. London: Routledge.
BLUMER, Herbert (1969) Symbolic Interactionism: Perspective and Method. Berkeley: University of California Press.
CALVIN, William H. (1983) The Throwing Madonna: Essays on the Brain. New York: McGraw-Hill.
DANIELSON, Peter (1992) Artificial Morality: Virtuous Robots for Virtual Games. London: Routledge.
DRAZIN, P.G. and R.S. JOHNSON (1989) Solitons: An Introduction. Cambridge: Cambridge University Press.
DYSON, Freeman J. (1988) Infinite in all Directions: The 1985 Gifford Lectures. New York: Harper and Row.
EDELMAN, Gerald M. (1992) Bright Air, Brilliant Fire: On the Matter of the Mind. New York: Basic.
EISER, Richard (1993) Attitudes, Chaos and the Connectionist Mind. Oxford: Basil Blackwell.
FISHER, J.C. and R.H. PRY (1971) 'A Simple Substitution Model of Technological Change', Technological Forecasting and Social Change, vol. 3.
FUGLESANG, Andreas (1982) About Understanding: Ideas and Observations on Cross-Cultural Communication. Uppsala: Dag Hammarskjold Foundation.
GABORA, Liane (1996) 'Culture, evolution, and computation' in the Proceedings of the Second Online Workshop on Evolutionary Computation March 1996, Sponsored by the Society of Fuzzy Systems and Theory, Nagoya University
GELL-MANN, Murray (1994) The Quark and the Jaguar: Adventures in the Simple and the Complex. New York: Freeman.
GLEICK, James (1988) Chaos: Making A New Science. New York: Penguin.
GORDON, Milton M. (1964) Assimilation in American Life. New York: Oxford University Press.
HARTH, Eric (1991) The Creative Loop. How the Brain Makes a Mind. Reading, Mass: Addison-Wesley.
HAWTHORN, Geoffrey (1991) Plausible Worlds: Possibility and Understanding in History and the Social Sciences. New York: Cambridge University Press.
HOMANS, George Caspar (1964) 'Bringing Men Back In', American Sociological Review, vol.29, pp. 804 - 818.
HUNTER, Floyd (1953) Community Power Structure: A Study of Decision Makers. Chapel Hill: University of North Carolina Press.
KAUFFMAN, Stuart (1995) At Home in the Universe: The Search for the Laws of Self-Organization and Complexity. New York: Oxford University Press.
KNUTH, Donald E. (1969) The Art of Computer Programming (vol. 2: Seminumerical Algorithms). Reading, Mass: Addison- Wesley.
LANGTON, Christopher G. (editor) (1995) Artificial Life: An Overview. Cambridge, Mass: MIT Press.
LEVY Jr., Marion J. (1968) 'Structural-Functional Analysis' in David L. Sills (editor) International Encyclopedia of the Social Sciences, Vol. VI, pp. 22 - 23. New York: Crowell, Collier and MacMillan.
LYNCH, Aaron (1998) 'Thought Contagion as Abstract Evolution', Journal of Ideas, vol. 2, no. 1, pp. 3 - 10. Reproduced at <http://www.mcs.net/~aaron/Lynch1991.htm>.
McNEILL, Daniel and Paul FREIBERGER (1993) Fuzzy Logic: The Discovery of a Revolutionary Computer Technology and how it is Changing Our World. New York: Simon and Schuster.
MARCHETTI, Cesare (1980) 'Society as Learning System: Discovery, Invention and Innovation Cycles Revisited', Technological Forecasting and Social Change, vol. 10.
MARQUETTE, Jesse F. (1981) 'A Logistic Diffusion Model of Political Mobilization', Political Behavior, vol. 3, no. 1.
MARSDEN, Peter V. and Nan LIN (1982) Social Structure and Network Analysis. Beverly Hills: Sage.
MISNER, Charles W.,Kip S. THORNE and John Archibald WHEELER (1973) Gravitation. San Francisco: W. H. Freeman.
NAGEL, Ernest (1961) The Structure of Science: Problems in the Logic of Scientific Explanation. New York: Harcourt, Brace and World.
NEWTON, Roger G. (1993) What Makes Nature Tick? Cambridge, Mass. Harvard.
PIPES, Richard (1995) Three Whys of the Russian Revolution. (The Barbara Frum Lectureship). Toronto: Vintage.
POLKINGHORNE, John (1986) One World: The Interaction of Science and Theology. London: SPCK.
RINALDI, S., G. FEICHTINGER and S. WIRL (1994) 'Corruption Dynamics in Democratic Systems', Research Report #168, Institute for Econometrics, Operations Research and Systems Theory. Technical University of Vienna. Vienna, Austria.
ROGERS, E.M. and D. Lawrence KINCAID (1981) Communications Networks: Toward a New Paradigm for Research. New York: Free Press.
ROSSER, J Barkley Jr (1991) From Catastrophe to Chaos: A General Theory of Economic Discontinuities. Boston: Kluwer.
SAVAGE, Adrian (1988) An Introduction to Chaos Magick. New York: Magickal Childe.
SAYADI, M.K. and J. POUGET (1991) 'Soliton Dynamics and Chaos Transition in a Microstructured Lattice Model' in Ioannis Antoniou and Franklin J. Lambert. (editors) Solitons and Chaos. Berlin: New York: Springer-Verlag.
SCHEFFLER, Samuel (1982) The Rejection of Consequentialism: A Philosophical Investigation of the Considerations Underlying Rival Moral Conceptions. Oxford: Clarendon Press.
SCHUSTER, Heinz Georg (1995) Deterministic Chaos: An Introduction (3rd Edition). Weinheim: Verlagsgesellschaft.
SKOCPOL, Theda (editor) (1984) Vision and Method in Historical Sociology. Cambridge: Cambridge University Press.
TEZUKA, Shu (1995) Uniform Random Numbers: Theory and Practice. Boston: Kluwer.
WELLMAN, Barry and S.D. BERKOWITZ (editors) (1988) Social Structures: A Network Approach. New York: Cambridge University Press.