Copyright Sociological Research Online, 1999

Jennifer Jarman, Robert M. Blackburn, Bradley Brooks and Esther Dermott (1999) 'Gender Differences at Work: International Variations in Occupational Segregation'
Sociological Research Online, vol. 4, no. 1, <http://www.socresonline.org.uk/4/1/jarman.html>

Abstract

Despite the prominence of discussions of gender segregation in explanations of labour market inequalities, there have been relatively few cross-national studies due to a lack of suitably detailed data. A recent ILO initiative obtained suitable data for cross-national analysis of 38 countries, with a much greater number of occupational categories than has usually been available. This paper reports findings from the analysis of these data. The problems and potential of using such data are discussed and a standardisation is introduced to control for the effects of the number of occupations in the segregation measure. There are important differences in the level of segregation in different countries. The highly segregated countries are to be found in Western Europe, and in particular Scandinavia. Several Arab countries also have high levels of segregation. An argument is made suggesting that the context and meaning of segregation patterns may be quite different from what might be inferred from single country studies.

Keywords:

Census/Survey Data; Cross-National Trends; Employment; Employment Patterns; Gender Inequality; Methodology; Occupations; Social Division of Labour; Sociology; Work

Introduction

1.1

Occupational gender segregation and its significance have been at the heart of debates about gender inequality over the last thirty years. It is widely accepted that segregation has a significant negative impact on the occupations and wages of women in comparison with those of men (Bradley, 1989; Reskin and Roos, 1990). Its study also gives important insights into the nature of women's broader integration in the social world outside the home and into the division of labour in society.

1.2

Despite the amount of sociological interest in the impact of globalization and its consequences for work and workers, and despite the importance placed by feminists on the generation, dissemination and analysis of gender-specific statistics^[1], there has been relatively little recent cross-national research on occupational gender segregation patterns and how these are affected by recent global economic and social processes. Not much attention has been paid to the effects of such things as shifts in the location of industries, telecommunications, migration of workers, changing citizenship boundaries, and different 'gender regimes' (Connell, 1987).

1.3

This has partly been due to the lack of appropriate data. A recent International Labour Office initiative has gone a small way towards rectifying this situation, and provides some baseline data which can be used as a starting point for understanding these issues. This paper presents a brief assessment of this data set, and explores the segregation trends and concentration patterns within it^[2]. Unfortunately the data set is quite limited and so many of the kinds of questions that an analyst would like to explore further remain elusive. Nevertheless, it does allow us to draw some tentative conclusions, particularly for the industrialised countries.

1.4

One of the most useful aspects of quantitative research is to provide a broader context in which to locate observations that have been made within a smaller frame of reference. With respect to segregation research, cross-national research allows us to pose a number of important questions: are levels of gender segregation high in all countries (as often appears to be assumed)? if not, which countries are highly segregated? which countries have low levels of segregation? what is the shape of the distribution of segregation levels across countries? are there any characteristics which are common to countries with similar levels of segregation? The answers to these questions take us beyond current understandings drawn from research based solely in national studies, and so lead us to a more adequate theoretical perspective on gender relations in employment.

1.5

We introduce a procedure to standardise for the number of occupations in each national data set, thus enabling proper comparisons across countries. Following on from this comparative analysis, we suggest not only that segregation may have very different meanings and consequences in different countries, as a few recent writers have noted (Rubery and Fagan, 1995), but that - contrary to previous assumptions - segregation may in fact enhance women's opportunities in certain situations.

1.6

If we are to understand gender segregation in employment, and associated patterns of inequality, we need to be able to look beyond the confines of national boundaries. Only by recognising and explaining the different patterns in different places can we reach an adequate understanding. The first requirement is a sound methodological approach in exploring the data, and so we pay considerable attention to this. We examine differences across countries in the levels of segregation and patterns of gender concentration, and see that they are quite substantial.

1.7

This leads us to the question of why do these differences exist. Naturally we cannot supply comprehensive answers without more research - perhaps others will take up the challenge and build on some of the work that has been done on segregation (e.g. Charles and Buchmann 1994; Charles 1992; Roos 1985). However, we are able to draw some interesting conclusions. An obvious factor to consider is the level of women's participation in the labour market, as greater participation may be expected to lead to a wider spread of their employment and so less segregation. This is not so; we find that high female participation is often associated with a labour force highly polarised into 'male' and 'female' occupations, though this is not sufficient to produce a relationship in the opposite direction (i.e. greater segregation). The patterns of concentration range from highly polarised Finland where almost two thirds of men and women work in occupations where at least 90% are their own sex, to Romania where 65% of women and 70% of men all are in one occupation (though we suspect this reflects ideology in occupational definition rather than social reality). The egalitarian countries of Scandinavia are among the most segregated, contrary to what one would expect on the basis of usual approaches or even common-sense. This leads to an appreciation of the fact that high levels of segregation , by enhancing female career prospects, may reduce gender inequality.

Literature Coverage

2.1

While on the face of it, the study of occupational gender segregation looks like a fairly well established area of second-wave feminist research and activity, on closer inspection it is not so well established as one would expect. The field has been relatively well served in terms of exploration of the dynamics of change within particular occupations in Europe and North America (Bradley, 1989; Reskin and Roos, 1990). There have been important contributions which attempt to theorize these dynamics with greater generality (e.g. Walby, 1988; Crompton and Sanderson, 1990). There are a few countries where there have been extensive programs of research - e.g. America (Jacobs and Lim, 1992; Reskin, 1993; Reskin and Roos, 1990; England, 1981); Great Britain (Hakim, 1979, 1992, 1993a, 1993b, 1996; Siltanen, 1990; Siltanen et al, 1995; Blackburn et al, 1993); and Europe (Rubery and Fagan, 1993, 1995). There are other countries where there have been a few important contributions but less systematic research programs. Finally, there are large sections of the world where there has been little or no research. In many countries of the 'South' a reverse situation exists to that in the 'North', in that national studies on employment patterns of women drawn from census and labour force surveys are more numerous than case study material for developing countries (Anker and Hein, 1986)^[3]. Even so, coverage and analysis is far less than is desirable^[4].

2.2

Some studies have attempted to make comparisons of national segregation levels across a number of countries (e.g. Charles 1992; Jacobs and Lim, 1992; Blau and Ferber, 1992). However they are based on very broad occupational classifications, typically using less that ten categories, which we have argued elsewhere is extremely unreliable (Siltanen et al, 1995).

Background

3.1

As part of its ongoing commitment to 'strengthen its advisory services and operational activities aimed at promoting equality of treatment for working women', the International Labour Office initiated a project titled the 'Interdepartmental Project on Equality for Women in Employment' which took place during 1992-1993. One of its activities was to commission a book which would clarify concepts and measures of occupational gender concentration and segregation and discuss measurement problems linked with the quality and availability of data (Siltanen et al, 1995). Another activity was to commission and facilitate the compilation of an international data set which could be used to analyse gender segregation in member countries of the United Nations. Census bureaux were contacted in all member nations of the United Nations and asked to provide the number of male and female workers by occupation in each country for three points in time: 1970, 1980 and 1990. Forty countries responded, although only thirty-eight had complete data coded in more than fifteen occupational categories. We have only analysed countries with data presented in twenty occupational categories or more. Geographical representation is uneven, however: only five African countries, two Latin American and two Caribbean countries provided data in contrast with nine Asian countries, eighteen European countries and two North American countries, Australia and New Zealand. These data have now been made available for analysis, and this paper reports some findings from their analysis. For various reasons we restrict the analysis to 35 countries, and generally 32. The effect is to increase the bias toward the developed countries of Europe, North America and Australasia.

Assessment and Discussion of the Data

4.1

The fact that this data set is drawn from national statistics and official sources has both positive and negative aspects. Clearly one has more confidence in the quality of data coming from countries where the national source is well respected in terms of quality of data collection, data cleaning, and an arm's length relationship to the political arms of government. In those countries where the national source is less well respected, 'official' status may guarantee nothing at all. It is important to be alert to the possibility that the data has been influenced by the governments in a manner consistent with the image which they would like to present to the world of the nature of their labour markets and gender representation within them. As might be expected, most of the countries supplying usable data were the ones with high quality statistical provision, thus limiting this problem.

4.2

It must also be recognized that these statistics pertain primarily to the formal sector. The International Standard Classification of Occupations (ISCO) does contain occupational categories such as 'Street Vendor' which are more pertinent to an assessment of informal sector activities, but informal sector activities have not been well captured in official data for a host of reasons^[5]. Therefore, economic activity which may be vital to provide large numbers of men and women with their livelihoods may not be captured by these data. This is more likely to be a significant problem in some countries in the sample - such as Mexico or Egypt - than in others, although informal activity exists throughout the sample. Since it has been estimated that women account for a high proportion of informal activity throughout the world, there is an inherent under-representation of women in official labour statistics. Again this is a less acute problem for most of the countries analysed here, both for countries supplying data and particularly for those retained for analysis; Mexico is eliminated though Egypt remains.

4.3

Comparing countries is a task fraught with problems about the meaningfulness of the comparisons being drawn. Can China, a country with one-fifth of the world's population, and practically a 'world region' in itself, be meaningfully compared to tiny Bahrain, a country of just over half a million people? Partly because of the enormous variations in size, nation-states are somewhat unsatisfactory units for sociological analysis. There are also patterns of cultural diversity which cut across national boundaries.

4.4

On the other hand, their political and administrative boundaries make them a reasonable choice. Laws relating to employment and to gender are generally determined nationally. Supra-national constraints, as for instance in Europe, may create similarities but do not undermine the appropriateness of national units of analysis. Economies tend to be structured nationally, and this has direct relevance for employment patterns. Multi-national corporations may be contributing to a growing trend of globalisation but this can be easily exaggerated. They adapt policies to local circumstances, often locating plants on the basis of the labour supply, such as cheap female labour in poor countries. Furthermore, in discussions of cultural diversity within countries, fundamental cultural similarities, like common language(s) tend to be taken for granted while diversity is apt to be emphasised. As in all research, there are no perfect units of analysis and ultimately adequate theoretical explanations must account for their diversity. However, while the fact that statistics are collected nationally may impose a constraint on our research, we should recognise that this constraint does reflect - if imperfectly - socio-economic reality.

4.5

In this data set, there are a number of different classification systems in use. Developing countries have tended to use the International Standard Classification of Occupations. There are two versions of ISCO represented in the data - ISCO68 and ISCO88 - depending upon what year the scheme was revised, and what year the country adopted a new scheme. Other countries have reported data using their own labour force or census occupational categories. Countries which have used the same ISCO schemes can be assumed to have higher levels of comparability though even here not all possible categories are included. Similarly, European Community countries have been moving towards similar systems of classification with the Labour Force Surveys and hence can be more meaningfully compared.

4.6

Some countries use much finer occupational divisions than others, thus identifying more occupations. The data for the United States of America and several European countries have in excess of four hundred occupational titles, and for Bahrain there are more than one thousand. The data for Romania and Iran, on the other hand, are presented at a much more aggregated level, with twenty and twenty-four occupational categories respectively^[6]. Even within a single country the occupational structure changes over time, and the classification scheme is revised. Nevertheless, there is a general consistency in the representation of occupational structures; the finer divisions are similar across countries and the broader categories may be regarded as groupings of the finer ones.

---

Table 1 : Countries Ordered by Degree of Segregation

---

	MM	Male Workers	Female Workers	F%*	#Occs	Year
Bahrain	.689	177154	34916	16.46	1050	1991
Kuwait	.672	532805	129783	19.59	282	1985
Finland	.661	1288900	1177200	47.74	478	1990
Norway	.638	1173733	970456	45.26	490	1990
UK	.635	14706170	11262018	43.37	526	1990
Costa Rica	.606	703812	293337	29.42	60	1991
Australia	.602	4583813	3241230	41.42	283	1990
Sweden	.601	2292400	2129400	48.16	52	1991
Cyprus	.601	127539	75536	37.20	383	1989
Angola	.600	262000	184000	41.35	71	1992
Switzerland	.595	1973757	1117937	36.16	541	1980
France	.584	12808000	9425000	42.39	454	1990
USA	.583	55899000	49334000	46.89	488	1991
Jordan	.570	376524	29540	7.28	80	1979
Hungary	.561	2513659	2013498	44.48	126	1990
Luxembourg	.552	104823	57451	35.40	78	1991
Mauritius	.535	282996	123647	30.41	386	1990
New Zealand	.528	879747	605223	40.76	305	1986
Poland	.522	9947632	8292286	45.46	373	1988
Austria	.520	2069200	1449500	41.19	77	1990
Canada	.516	6231000	5257000	45.74	41	1990
Spain	.498	8576200	4003100	31.82	82	1990
Hong Kong	.483	1686000	1029000	37.90	78	1991
Tunisia	.482	1721000	416400	19.48	59	1989
Haiti	.481	1068000	776000	42.08	70	1986
Japan	.455	3733113	2440083	39.53	294	1990
Bulgaria	.452	2507000	2179000	46.50	47	1985
Iran	.450	8664000	929000	9.68	24	1986
Italy	.431	14244172	6787138	32.27	249	1981
Egypt	.420	10098399	988296	8.91	80	1986
Malaysia	.316	2903341	1365334	31.98	80	1980
Korea, Rep of	.308	10686000	6408000	37.49	44	1989
Senegal	.169	1746555	601001	25.60	88	1988
Romania	.159	4633433	3272225	41.39	20	1990
China	.151	293661000	227844000	43.69	302	1980

---

*F% is the percentage of the labour force which is female.

4.7

In order to achieve consistency, the analysis of segregation and patterns of concentration was restricted to members of the employed labour forces in the different countries, and excluded unemployed workers^[7]. Workers whose occupations are not specified and occupations listed as 'Not Elsewhere Classified', have been dropped as they tend to be products of the coding scheme rather than distinct occupations.

Gender Segregation

5.1

The level of segregation in each country is measured by the marginal matching coefficient, MM. This is a measure of the extent to which men and women are polarised into 'male' and 'female' occupations. It ranges from 0 (no segregation) to 1 (complete segregation). At one extreme, if there were only one occupation there could be no gender segregation, and it would also be zero where all occupations comprised the same 'expected' proportions of men and women. At the opposite extreme, if every person had their own unique occupation or in any other situation where all occupations were staffed by only women or by men (as would occur in a labour force comprised solely of monks and nuns) there would be complete segregation. In practice, of course, measured segregation lies between these extremes.^[8] Table 1 presents the values of MM for the data as supplied for the various countries, together with the number of occupations, the gender distribution of workers and the relevant date (the most recent supplied). The countries are ordered according to their levels of measured segregation.

Controlling for the Number of Occupations in Measuring Segregation

6.1

All estimates of segregation are affected by the number of occupations included in the analysis. The value of MM (or any other segregation measure) increases with the number of occupations used in the measurement. When occupations are combined into a larger category, not only is the diversity of gender ratios replaced by one ratio but there is a form of regression to the mean, so the 'expected' level of measured segregation varies with the number of occupational categories. Each observed national segregation level may be regarded as the expected level for that number of occupations together with the national deviation from the expected value - the standard situation of regression analysis. Since there were wide variations across countries in the numbers of occupations, this had a substantial effect on the apparent differences in national segregation levels. In previous research no attempt to control for this effect has been made. Indeed this is hardly surprising as a suitable data set has not been available. So, in order to make more accurate comparisons across countries, we decided to measure the effect on the observed value of MM of the number of occupations in a data set. We then standardised for each country on 200 occupations.

6.2

To do this, we estimated an equation to relate the 'expected' value of MM to the number of occupations, using the set of data in Table 1 and several additional national data sets (See Appendix for details). Of course the actual 'observed' value of MM for each country differed from the estimated 'expected' value for the number of occupations in the data set for that country. For each country we calculated the observed to expected ratio. Then we applied this ratio to the estimated value for 200 occupations^[9]. This gave us a set of comparable estimates of segregation level for a notional set of 200 occupations in each country.

6.3

Our initial estimating equation was:

where n is the number of occupations and , 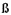and y are the three parameters that are possible in the estimation equation^[10].

However, it turned out that the estimate of y was approximately 1 in all the equations providing a good fit. We therefore dropped it from our estimating procedure and used the simpler equation.

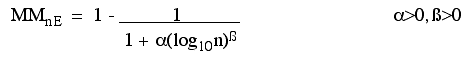
We estimated the optimal values are = 0.60 and 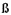= 0.93.

Thus the final equation arrived at was

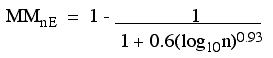
(For further details of the derivation of this formula see the Appendix).

6.4

Applying this formula to the countries of Table 1, and calculating the values for MM_200E we obtain the revised, standardised values of MM shown in Table 2 as MM₂₀₀. As we would expect the ordering of countries is not vastly different, the Spearman rank correlation being 0.85. Inevitably, there is some convergence as MM is decreased in countries recording more than 200 occupations and is increased in countries recording less than 200. What is important is that now we can make direct, meaningful comparisons across countries. As in all forms of statistical analysis, each measurement is liable to contain an element of error, so that countries with similar values on MM₂₀₀ may possibly be wrongly ordered. However, these are the best comparative measures available and, with appropriate caution, will be treated as accurate.

---

Table 2 : Standardised Segregation Measure, MM₂₀₀ and Unstandardised MM

---

	MM200	Rank	MM	Rank
Sweden	.683	1	.601	8
Costa Rica	.677	2	.606	6
Angola	.658	3	.600	10
Kuwait	.655	4	.672	2
Finland	.623	5	.661	3
Bahrain	.622	6	.689	1
Jordan	.618	7	.570	14
Canada	.604	8	.516	21
Norway	.601	9	.638	4
Luxembourg	.600	10	.552	16
UK	.595	11	.635	5
Australia	.587	12	.602	7
Hungary	.583	13	.561	15
Cyprus	.574	14	.601	9
Iran	.569	15	.450	28
Austria	.566	16	.520	20
Switzerland	.557	17	.595	11
France	.552	18	.584	12
USA	.548	19	.583	13
Tunisia	.540	20	.482	24
Spain	.538	21	.498	22
Haiti	.528	22	.481	25
Hong Kong	.525	23	.483	23
Bulgaria	.520	24	.452	27
New Zealand	.512	25	.528	18
Mauritius	.511	26	.535	17
Poland	.500	27	.522	19
Egypt	.455	28	.420	30
Japan	.443	29	.455	26
Italy	.424	30	.431	29
Korea, Rep of	.357	31	.308	32
Malaysia	.343	32	.316	31
Romania	.207	33	.159	34
Senegal	.181	34	.169	33
China	.147	35	.151	35

---

6.5

Comparing Table 2 with Table 1, we see that the most dramatic changes in ranking involve Canada and Iran, which both go up 13 places. Sweden and Angola each rise 7 places, putting them in first and third positions on MM₂₀₀, while Jordan has a similar rise from the 14th to the 7th highest segregation level. Countries with the greatest descent in the rankings are Mauritius, Poland and New Zealand, dropping 9, 8, and 7 places respectively.

Differences Across Countries

7.1

We see from Table 2 that there is quite a wide range of differences, with standardised segregation ranging from 0.147 in China to 0.683 in Sweden. There is no theoretical basis on which we can designate countries as having high or low segregation. In practice there seems to be an assumption that all countries have high segregation; at least it is very difficult to find any reference to countries with low segregation.

7.2

Since we know that gender is a significant factor in the organisation of virtually all societies, we would expect to see evidence of this when we look at labour market patterns. Our criterion for a country with a 'low' degree of segregation is simply that it has a relatively low value on our measure of segregation. Similarly, a 'high' level of segregation describes a country which has a relatively high value. The consequence of this way of proceeding is that there is then a point to doing cross-national investigations. Rather than assume that every country has a high level of segregation, we can then think about the patterns in contrast to one another. This makes the countries with 'high' levels seem more exceptional, as well as the countries with 'low' levels.

7.3

In looking at the segregation figures it is interesting that they are quite evenly spread. Although the difference is greater than 0.5 over the total range, the differences tend to be concentrated at the bottom of the rankings. From Sweden, at the top, to Poland in 27th position the difference is less than 0.2. There is a fairly even gradual decline over this range, the only notable gap being between Kuwait at 4th with 0.655 and Finland in 5th position with an standardised MM of 0.623.

7.4

Highly segregated occupational structures may be pragmatically defined as those with MM₂₀₀ values of 0.6 or more. These include the three Scandinavian countries: Sweden, Finland and Norway. It is interesting that these are countries noted for policies and practices of gender equality. Canada may also be regarded as a relatively egalitarian country with respect to gender. On the other hand, the highly segregated also include Arab countries which are not known as leading practitioners of gender equality.

7.5

It is much harder to be clear about countries with low levels of occupational segregation. The three that emerge as the ones with the lowest levels are somewhat misleading. Romania (.207), China (.147) and Senegal (.181) all appear to have exceptionally low segregation, but in each case this is a consequence of most of the workforce being classified to the same occupation. Romania, for example, has two thirds of its labour force classified as production workers. Since this is a very diverse category which covers numerous groups in other national schemes, little meaning can be given to comparisons between this value of MM₂₀₀ and the results for other countries. (Recall that it is also a country with a high level of aggregation in the data - only 20 groups - which tends to further undermine comparability.) Senegal has 59% of its workers employed as 'agricultural workers'. While this is a meaningful and fairly coherent category, suggesting a genuine situation of low segregation, it could easily be subdivided. Similarly in China, 'grain farmers' constitute 66% of the total labour force, even though 301 other occupations are identified. We are advised that the category is far more diverse than the name suggests. Excluding this category, the segregation measure rises to 0.415, indicating a low to moderate level of segregation; probably a realistic estimate would lie between the two values and so be quite low. In order to maintain a reasonable level of comparability across countries, these three will not usually be included in the further analysis of segregation levels.

7.6

Two other countries emerge as having particularly low segregation, South Korea (.357) and Malaysia (.343). In these countries the measures are not so strikingly low but seem to be reasonably unproblematic. Not surprisingly, agriculture features quite prominently in both South Korea and Malaysia; in South Korea, farming is a single category (out of 44) accounting for nearly a quarter of the labour force and in Malaysia there are two farming categories which together make up 36%, but these large groups seem to be realistic.

Segregation Level and the Female Share of Employment

8.1

We might expect that the relationship between the female share of employment (F%) and segregation level would help us to understand upon what terms women's participation is guided. We might well expect that as more women enter the labour force they are to be found in a greater breadth of occupations, and therefore less segregated from the men. This is not what we find. Of the countries with the lowest female participation levels, Jordan and Bahrain^[11] do have relatively high levels of segregation, which means that a large part of the labour force comprises men in men-only jobs. In contrast, however, the low level of female participation in Egypt is combined with a relatively low level of segregation. This means that those women who do participate in the labour force are fairly well dispersed across occupations rather than concentrated in a few. At the other extreme with the highest level of female participation is Sweden, which has not the lowest but the highest level of segregation. While the results for several countries are quite striking and contrasting, the overall pattern is one of no relationship between female participation levels and segregation.

8.2

If the female share of the labour force has no bearing, perhaps its absolute size does. Obviously this is related to the size of the country's entire labour force, but the hypothesis is that where there are fewer women they are more likely to be found in just a few occupations. The more numerous men, on this argument, would be more widely spread. At first sight there appears to be a modest trend in this direction. However, despite the varying gender ratios, the trend seems just as clear for men. This points to the conclusion that smaller countries, in terms of employed populations, tend to have higher levels of gender segregation. It is not clear why this is so.

8.3

It might be thought that industrialisation is related to the segregation level. If so, this might well explain the lower segregation of the labour force in larger countries. However, there is no clear pattern relating segregation levels and industrialisation. With a larger sample this might emerge. However, it seems likely that two contrary trends are operating. On the one hand, there may be a trend towards greater integration in the more industrial countries, with women entering former 'male' occupations; on the other hand, greater occupational diversity and specialisation may increase the degree of segregation.

8.4

Another way in which we may look for explanations is to compare regions of the world, though conclusions must be tentative because of the thin coverage in most regions. The interest is not, of course, in the physical locations but in cultural patterns; for instance, political and religious influences are potential sources of differences in the gender patterns of employment.

8.5

Table 3 presents the extremes of high and low segregation and the median country for some loosely defined regions. We see that the countries of Western Asia have high levels of segregation while the rest of Asia has notably low levels. In Europe there is generally a high level of segregation in the West and a lower level in the East. While some general differences are apparent, perhaps the most significant result is the pattern of wide differences in almost every region.

8.6

Within Europe it is the Scandinavian countries which stand out as having particularly high levels of segregation. On the face of it, this is rather surprising. These countries are well known for being exceptionally egalitarian, and this reputation includes gender. Making sense of this leads us to focus on the vertical and horizontal dimensions of segregation, where the vertical is the direct measure of inequality and horizontal measures difference without inequality.^[12] We hypothesise that in the Scandinavian countries, unlike some other highly segregated countries, the observed segregation level is made up of a large horizontal component and a small vertical one (Blackburn and Jarman, 1997).

8.7

More generally, we suggest that there is an inverse relation between segregation and its vertical (inequality) component. This may be counter-intuitive and contrary to general assumptions, but a little consideration will show it does make sense. Where men and women compete for better jobs, the evidence is that the jobs more often go to men. Consequently, the greater the degree of segregation, the higher are women's promotion prospects and the more equal is the outcome (Blackburn, Jarman and Brooks 1999). While this makes sense of the observed data and fits the pattern of change in Britain (Blackburn, Brooks, and Jarman 1999), thorough testing must wait on further research.

---

Table 3 : Segregation of Countries by Region: Lowest, Median, and Highest Values

---

Lowest	Median	Highest
DEVELOPED COUNTRIES
W. Europe
Italy (.424)	Austria (.566)	Sweden (.683)
E. Europe
Poland (.500)	Bulgaria (.520)	Hungary (.583)
Other
Japan (.443)	USA (.549)	Canada (.604)
AFRICA
Egypt (.455)	Tunisia (.540)	Angola (.658)
LATIN AMERICA AND THE CARIBBEAN
Haiti (.529)		Costa Rica (.677)
ASIA AND THE PACIFIC
E., S.E., and S. Asia
Malaysia (.343)	Rep. Of Korea (.357)	Hong Kong (.525)
W. Asia
Iran (.569)	Jordan (.618)	Kuwait (.655)

Note: Romania, Senegal and China are excluded from this table; if included they would each have the lowest value for the relevant group.

---

Gender Concentration

9.1

Patterns of female concentration (the percentages of the workers in occupations who are women) are useful to help illuminate an understanding of the underlying features of the data which contribute to the overall trends. There are several main concentration patterns, though they are not entirely distinct and in some countries two patterns merge. Almost all the countries have one feature in common: a substantial number of men working in occupations staffed entirely or almost entirely by men. The extent of this varies between the patterns, and not all patterns show a corresponding grouping of women in exclusively female occupations.

Figure 1: Number of Workers by Level of Female Concentration (percentage of workers in an occupation who are women, with the range from 0 to 100 divided into 10 equal groups)

Pattern 1 examples
1. Finland 1990

    Count   Midpoint
  5871000       5.00 _______________________________________________
  2895000      15.00 ________________________
   954000      25.00 ________
  2444000      35.00 ____________________
  1035000      45.00 _________
  1270000      55.00 ___________
  1345000      65.00 ____________
  1698000      75.00 ______________
  1221000      85.00 ___________
  5949000      95.00 _______________________________________________
                     I....+....I....+....I....+....I....+....I....+....I
                     0        1280       2560      3840      5120    6400
                                      Number of workers (thousands)


2. UK 1990                               
 
    Count   Midpoint
  7058353       5.00 _____________________________________________
  2601457      15.00 _________________
  1582321      25.00 ___________
  2448438      35.00 ________________
  1088880      45.00 ________
   556013      55.00 ____
  2010840      65.00 ______________
  4908623      75.00 ________________________________
   573218      85.00 _____
  3140052      95.00 _____________________
                     I....+....I....+....I....+....I....+....I....+....I
                     0       1600      3200      4800       6400    8000
                                      Number of workers (thousands)

9.2

The first pattern is found only where a fairly substantial proportion of the labour force are women. It consists of an occupational structure which is polarized along male and female lines. Finland provides the most clear cut example, with many occupations that are strongly male-dominated and others that are strongly female-dominated. There are not many occupations which are fairly evenly mixed. This is a fairly common pattern, and other countries with similar, if a little less obvious, polarised gender structures include Sweden, Norway, the United Kingdom, Haiti, and the USA. The pattern is associated with moderate and high segregation. Despite the polarised pattern, these are not all among the most segregated countries; in fact they are fairly widely dispersed through Table 2.

Figure 2: Number of Workers by Level of Female Concentration (percentage of workers in an occupation who are women, with the range from 0 to 100 divided into 10 equal groups)

Pattern 2 examples
1. Malaysia 1980  

    Count   Midpoint
   934474       5.00 ______________________________
   325783      15.00 ___________
   292564      25.00 __________
  1216912      35.00 _______________________________________
  1131809      45.00 ____________________________________
   126562      55.00 _____
    33503      65.00 __
   129749      75.00 _____
        0      85.00 _
    77319      95.00 ___
                     I....+....I....+....I....+....I....+....I....+....I
                     0        320       640       960       1280    1600
                                      Number of workers (thousands)
2. Bahrain 1991   

    Count   Midpoint
   141997       5.00 _____________________________________________
    20527      15.00 _______
    11197      25.00 ____
     4759      35.00 __
     3568      45.00 __
     1323      55.00 _
     6025      65.00 ___
     4382      75.00 __
    17507      85.00 ______
      785      95.00 _
                     I....+....I....+....I....+....I....+....I....+....I
                     0        32        64        96        128      160
                                      Number of workers (thousands)

9.3

A second pattern is found where women's participation in employment is moderate or low. In this pattern there are a number of male-dominated occupations, some mixed, but few or no female occupations. Whatever work women do, they are likely to be in the minority. Egypt, Senegal, Malaysia, Mauritius, and Mexico display this pattern, although we have some doubts about the data for Senegal (one large category) and Mexico (too few categories). Bahrain blends this with the first pattern, having too small a female labour force for that pattern. Apart from Bahrain, this is a pattern of fairly low segregation.

9.4

A third pattern is an occupational structure which has peaks at the extremes in terms of almost entirely male occupations and almost entirely female occupations, with a strong representation in the mixed occupations as well. Thus, it differs from the first pattern by the large number of women and men working in mixed occupations. Poland illustrates this pattern, as do Angola, Canada and Luxembourg. This appears to be another pattern associated with varying levels of segregation.

Figure 3: Number of Workers by Level of Female Concentration (percentage of workers in an occupation who are women, with the range from 0 to 100 divided into 10 equal groups)

Pattern 3 example
Poland 1988
 
    Count   Midpoint
  4364541       5.00 ______________________________________________
  1106794      15.00 _____________
  1019412      25.00 ____________
   676035      35.00 ________
   854910      45.00 __________
  4784504      55.00 ___________________________________________________
   562905      65.00 _______
   540594      75.00 _______
  2258429      85.00 _________________________
  2071794      95.00 _______________________
                     I....+....I....+....I....+....I....+....I....+....I
                     0        960       1920      2880      3840     4800
                                      Number of workers (thousands)

9.5

Sometimes, instead of the peak of almost entirely female occupations there are few workers in entirely female occupations, giving a fourth pattern. Workers tend to be grouped in male occupations or in ones where there are only a moderate proportion of women. Japan and Austria fit this pattern. France and Cyprus provide a variation on both these patterns, where the second peak is flattened, giving a fairly even spread of workers in the occupations with medium and high percentages of women workers. These are all patterns of medium or low segregation.

Figure 4: Number of Workers by Level of Female Concentration (percentage of workers in an occupation who are women, with the range from 0 to 100 divided into 10 equal groups)

Pattern 4 example
Japan 1990   
 
    Count   Midpoint
  1526606       5.00 _________________________________________________
   557920      15.00 __________________
   501238      25.00 _________________
   204464      35.00 _______
   571956      45.00 ___________________
  1489590      55.00 ________________________________________________
   497026      65.00 _________________
   358734      75.00 ____________
   282456      85.00 __________
   183206      95.00 _______
                     I....+....I....+....I....+....I....+....I....+....I
                     0        320       640       960       1280    1600
                                       Number of workers (thousands)


Pattern 4/3 example
France 1990
 
    Count   Midpoint
  5416089       5.00 ___________________________________________
  1648091      15.00 ______________
  2083088      25.00 _________________
  2312713      35.00 ___________________
  1748341      45.00 _______________
  1782120      55.00 _______________
  1623309      65.00 ______________
  1114771      75.00 __________
  2212450      85.00 __________________
  2292002      95.00 ___________________
                     I....+....I....+....I....+....I....+....I....+....I
                     0       1280       2560      3840      5120    6400
                                      Number of workers (thousands)

Figure 5: Number of Workers by Level of Female Concentration (percentage of workers in an occupation who are women, with the range from 0 to 100 divided into 10 equal groups)

Pattern 5 examples
1. Korea 1989                            
 
    Count   Midpoint
  2479000       5.00 ____________________
  1008000      15.00 _________
  1560000      25.00 _____________
  3992000      35.00 ________________________________
  5093000      45.00 _________________________________________
   463000      55.00 _____
  1824000      65.00 _______________
        0      75.00 _
   684000      85.00 ______
        0      95.00 _
                     I....+....I....+....I....+....I....+....I....+....I
                     0        1280      2560      3840       5120    6400
                                      Number of workers (thousands)
2. Romania 1990                          
 
    Count   Midpoint
   118344       5.00 __
   623276      15.00 ______
   245282      25.00 ___
  5367925      35.00 ___________________________________________
   157684      45.00 __
   247254      55.00 ___
   409492      65.00 ____
   681629      75.00 ______
    54772      85.00 _
        0      95.00 _
                     I....+....I....+....I....+....I....+....I....+....I
                     0        1280       2560      3840       5120    6400
                                      Number of workers (thousands)

9.6

The final pattern is an occupational structure predominated by mixed sex occupations. China, South Korea, Romania and Bulgaria are examples. We have already indicated our reservations about the classification schemes for China and Romania; indeed the illustration for Romania clearly shows the effect of the one massive category which has cast doubts on the low measure of segregation. However, even if we ignore this category, the concentration pattern has an interesting feature, compatible with low segregation; it is unusual in having few workers in the almost entirely male occupations. This is the reverse of the first pattern we considered, and is associated with low levels of segregation. However, it is like the first pattern in that there is a fairly high level of female participation in the labour force.

Occupational Patterns

10.1

Another way to look at concentration is in terms of women's representation in different occupational sectors of the labour force. For this purpose we have divided the occupational structure into six broad categories:

• Professional and technical
• Clerical and services
• Sales
• Manufacturing and transport
• Administration and management
• Agriculture, fishing and forestry

10.2

In Figure 6 we present the extent of female employment in these sections of the labour market, i.e. the percentage of the workers in each occupational grouping who are women. We do this for four countries.

10.3

The most striking feature is the similarity of all the patterns. Norway and Sweden are both included to show how very similar the patterns are for the two highly segregated Scandinavian countries. Japan and Mauritius are countries with quite different cultures, labour markets and levels of female participation. However, apart from relatively high employment of women in agriculture their patterns are much the same as the Scandinavian countries. We see, therefore, that the different levels of segregation in the countries are not due to the type of occupational distribution, in these broad terms. Only the relative extent of agricultural employment appears to be inversely related to segregation. Beyond this the determinants of segregation operate at the level of finer occupational divisions.

10.4

It should be noted that the scales are quite different, reflecting the different levels of female participation, or more precisely the differences in the highest concentration levels.

10.5

In all four countries the greatest concentration of women is in clerical and service work. Professional and technical work comes second except in Japan where it is a little behind agricultural employment. Sales is another area of relatively high female employment. On the other hand, Administration and Management have a low concentration of women in each country. The chief differences concern participation in manufacturing and agriculture. We have already noted that women's participation in agricultural work is lower in the two European countries, and in these countries their participation in manufacturing it is even lower. On the other hand, in Mauritius manufacturing has a relatively high level of employment for women. Mauritius has a lower overall rate of female participation than the developed countries and a more even spread of women across the six occupational types of employment.

Figure 6: Female Concentration Across Six Occupational Groupings

Japan 1990 
Occupation           Female percentage of workers

Professional, tech. 40 __________________________________
Clerical & services 58 _________________________________________________
Sales               37 ________________________________
Manufacturing, etc. 28 _________________________
Administration       9 ________
Agriculture, etc.   45 ______________________________________
                       I.........I.........I.........I.........I.........I
                       0        12        24        36        48       60
                        
Mauritius 1990          
Occupation	         Female percentage of workers
 
Professional, tech. 35 _____________________________________________
Clerical & services 37 _______________________________________________
Sales               27 ___________________________________
Manufacturing, etc. 29 ______________________________________
Administration      17 ______________________
Agriculture, etc.   27 ___________________________________
                       I.........I.........I.........I.........I.........I
                       0         8        16        24        32       40

Norway 1990
Occupation		   Female percentage of workers

Professional, tech. 57 ____________________________________  
Clerical & services 76 ________________________________________________
Sales               54 __________________________________
Manufacturing, etc. 15 ________
Administration      31 __________________
Agriculture, etc.   27 _________________
                       I.........I.........I.........I.........I.........I
                       0         16       32        48        64       80                         
Sweden 1991
Occupation           Female percentage of workers
                       
Professional, tech. 63 ___________________________________________
Clerical & services 73 _________________________________________________
Sales               55 ______________________________________
Manufacturing, etc. 18 _____________
Administration      34 _______________________
Agriculture, etc.   24 _________________
                       I.........I.........I.........I.........I.........I
                       0        15        30        45        60       75
   

10.6

There are far too many occupations at the more detailed level to do any comparable analysis. There are, nevertheless, some general points which are in line with the foregoing discussion. Some countries have a number of occupations with very high female concentrations (98% or more), eg Cyprus, France, Japan and Poland, and most have occupations where the female concentration is more than 90%. Where there are relatively few women in the labour force, strongly female-dominated occupations are inevitably rare, and Jordan, Egypt, Iran and Tunisia have no occupations with female concentrations more than 90%. The same is true of Costa Rica which has only moderate levels of female participation, but South Korea and Romania are exceptional among the countries with fairly high female participation in having no such markedly female occupations.

10.7

The occupations with the high female concentrations, and particularly the larger ones, are almost all in the 'clerical and services' or 'professional and technical' groups. Prominent in many countries are secretarial work, typing, nursing, care of young children, and domestic work as servants, cleaners etc. There are, of course, some distinctive national variations; for example, Japan has an all-female occupation of 'geisha and dancing partners'.

10.8

The similarities of these patterns (and those not shown) may seem surprising when we consider the variety of patterns of concentration levels we presented earlier. However, it is in line with our earlier observation that the occupational structures of the various countries are similar. It appears that at a basic economic level societies are similar. This is what we would expect in view of the widespread currency of technological and organisational ideas - an element of globalisation. On the other hand, gender ideologies and gender relations also depend on political, religious and general cultural factors which entail considerable diversity.

Conclusions

11.1

There are notable differences in the levels of segregation around the world. Analysis reveals, however, some very strange bedfellows amongst the countries which are found to be most highly segregated. Western Europe, and in particular, Scandinavia, stands out as being highly segregated. So too, do several Arab countries, specifically Bahrain and Kuwait. This suggests that the context and meaning of segregation patterns cannot be assumed. It may be the case that high levels of segregation can be a contributing factor in reducing gender-based inequalities. The positive consequences of segregation have been noted in some of the literature on girls' educational attainments. It is logical to think that there are also positive aspects that accrue to women working in female-dominated occupations. Such might include the creation of all-female job ladders such that supervisory and managerial positions are opened for women to supervise other women, the creation of female zones of influence which are protected from male applicants, female-friendly workplaces and work policies, women's trade unions. This clearly is an hypothesis which needs further development as it goes against much of the logic of second wave feminist action and thinking of the last twenty years (indeed it is closer in thinking with first wave feminist activity).

11.2

Generalisations about the countries which are the least segregated are more difficult to sustain as analysis reveals some serious problems in occupational coding of the countries that appear to be least segregated. Despite these problems, the data do suggest that countries with a large percentage of the labour force working in agriculture tend to be less segregated than the more industrial countries. Because of the coding problems this finding must be viewed as needing further analysis and might be a fruitful topic for detailed case study.

11.3

One might expect, on cultural and statistical grounds, that countries with high levels of female participation would be less segregated, but there is no such trend. Indeed, in many countries where women are a substantial part of the labour force they are not spread through the occupations but highly concentrated in a few, as for example in Finland. Industrialisation tends to be associated with greater involvement of women in the economy, including more active women's movements, which might also be expected to have other effects. However, it seems these effects pull in opposite directions; women are more integrated in the economy and so tend to enter 'male' occupations, while greater occupational diversity and specialisation leads to more segregation.

11.4

We found more diversity within than between regions of the world. This is not too surprising, as the regions were pretty broad. There is one pattern that does seem to emerge which is partially regionalised. It does appear that the communist (or former communist) countries have less segregation than the capitalist ones (even allowing for ideological bias in the statistics). Other political, religious and cultural factors appear likely to influence women's employment and gender segregation. However, these, and indeed all factors suggested as relevant, must be viewed as tentative hypotheses. Our broad overview may suggest interesting patterns, but more detailed research is needed to establish firm conclusions.

11.5

Occupational segregation is influenced by, and helps to maintain inequalities, not only at work but in a society. The actual significance will, nevertheless, vary depending upon the broader context in which they exist. Clearly part of the key to understanding this broader context is to focus not only upon overall segregation, or how women and men are distributed across occupations, as this paper has done, but also upon analysing vertical segregation, or how rewards such as pay and status are associated with occupational categories. Unfortunately, while the data set compiled by the International Labour Office provides a basic understanding of some relevant trends and patterns with respect to gender concentration and segregation it does not contain data which would allow for such an analysis. In the near future, the scope for large scale cross-national analysis of vertical segregation patterns is fairly limited. Indeed, it is clear that there is considerable work to be done to attain the goals of the Beijing Women's conference with respect to gender-specific statistics that are of good quality and that include sufficient variables for a thorough investigation of men's and women's labour market situations and that are accompanied by in-depth documentation concerning the collection and coding procedures.

Appendix

(i) Occupational Coding

Researchers have paid most attention to the question of consistency in occupational classification schemes. At the most basic level, differences in coding instructions and coding practices can affect the comparability of occupational data (see Hussmanns et al, 1990: pp. 173ff). Even when the coding schemes are the same there may be inconsistencies in the actual coding. Two equally important considerations are the ease with which the scheme can be used consistently, and the quality of the coding itself.

An important consideration is the consistency of detail within occupational groups. Some areas of employment may have been more finely broken down than others so that, for example, there may be several forms of service work identified but relatively fewer classifications for manufacturing work. This has implications for the measured levels of gender concentration and segregation. In this respect, it has frequently been observed that women tend to be clustered in a small number of occupations, in contrast to men who are spread across a much larger number. While this pattern may reflect disparities in the employment opportunities of women and men, it has often been suggested that it is partly a product of the occupational classification scheme. Since occupations that are predominantly male have received more attention from researchers and government statistical organizations, they have tended to be classified more finely. In contrast, women's occupations have tended to be classified in large umbrella categories. Attempts to construct an interpretation of gender inequalities in employment based on the more restricted distribution of the female labour force relative to that of the male labour force, such as discussions around the 'crowding' of women into a limited number of occupations, would clearly need to be tempered by an awareness of this problem. This aspect of classification may also help to explain why there has been no discussion of the 'crowding' of men, despite the very high male concentrations in some occupations. The striking version of this problem in the present data is the extremely high proportion of the workforce in a single occupational group - ranging from 59% to 67% - in three countries: Senegal, China and Romania. Inevitably all three show low levels of segregation, but it is doubtful whether these results are meaningful in comparison to others.

(ii) Key Concepts

While the term 'segregation' is currently in widespread use, there are inconsistencies in usage which have resulted in disagreements and misunderstandings. Differences in the use of concepts also have different consequences for decisions about data analysis. Here we present a brief account of the use of the concepts of 'segregation' and 'concentration'. For a fuller discussion see Siltanen et al (1995).

'Segregation' is used as a general term to include a number of different data patterns. All of these patterns relate to the distribution of men and women in the labour force, and all are of interest in the study of gender inequality. However, they are essentially of two distinct kinds (discussed below), and each kind carries with it its own set of methodological issues. One of these kinds of data patterns is always referred to as segregation, and we shall keep to this practice. Sometimes the second kind is also called segregation, but in order to be more precise in the analysis and discussion or gender patterns in employment, we argue that it is more appropriate to refer to it as concentration.

Segregation

Segregation concerns the tendency for men and women to be employed in different occupations from each other across the entire spectrum of occupations under analysis. It is a concept which is inherently symmetrical, entailing the relationship of women workers to men workers. In so far as women are separated from men, so are men separated from women in the labour forces under consideration. Men cannot be more 'segregated' than women, nor women more 'segregated' than men. Women and men are segregated in relation to one another and, therefore, both are segregated to the same degree.

A situation of total segregation would exist if all occupations were staffed exclusively by one sex or the other - that is, there were no occupations in which both men and women were employed. In some countries there are many single-sex occupations (particularly where the female share of the labour force is low) but there are also a substantial number of occupations where both men and women are employed. Nevertheless, all occupations are gendered in that they are, to differing degrees, predominantly male or predominantly female. Segregation refers to the extent to which this pattern occurs - the extent to which the sex distribution across occupations approaches total segregation. This may be conceptualised as the relationship between the gendering of occupations and the gender of workers. There would be no relationship, that is no segregation, if the mix of women and men in each occupation were the same. In practice this does not happen and there is always some degree of segregation. While there is a tendency for people to think of segregation as meaning total segregation, in fact there is a range of values that are relevant, and the level can vary - at least in principle - from zero segregation to total segregation.

Segregation Measures

There are a number of different possible choices for a measure of segregation. Debates over the criteria for making decisions about the validity of various indexes go back to the 1950s, when it was racial segregation that was the focus of attention. The measure of segregation which we use in this paper is based on Marginal Matching. Marginal Matching is a procedure developed by Robert M. Blackburn and Catherine Marsh (1991) when they were attempting to measure patterns of educational attainment by people from more and less advantaged home backgrounds. Its strength is that it responds consistently to changes in segregation without being affected by other aspects of changing circumstances (such as the increase or decrease of women in the labour market). A discussion of the strengths and weaknesses of this approach and of other indexes is provided in Siltanen et al (1995).

Concentration

Concentration actually encompasses a family of patterns with underlying features which are related. It is concerned with the sex composition of the workforce in an occupation or set of occupations. Whereas segregation refers to the symmetrical separation of the two sexes across occupations, concentration refers to the representation of one sex within one or a group of occupations, and so is necessarily not symmetrical. However, segregation may be understood as a measure that summarises the overall pattern of concentration.

The most widely used conception is the gender distribution within a particular occupation. Usually it is measured as the percentage of workers in the occupation who are women. This is a measure of the concentration of women in the occupation, and similarly the concentration of men is measured by the male percentage of workers in the occupation. For example, if 80 women and 20 men are employed in a particular occupation, the female concentration is 80% and the male concentration is 20%. While the usual practice is to measure the concentration of women, the male concentration is readily deducible from this.

The main point of interest is often the extent to which occupations are dominated by one sex, perhaps by identifying the two or three occupations with the highest concentrations of women, or the proportions of occupations employing each sex exclusively. From a broader perspective, the interest may lie in the general pattern of polarisation into predominantly female and male occupations. Although concentration typically measures the gender composition of an occupation, it can equally well apply to a group of occupations, an industry or a section of the labour force such as part-time workers. Whatever the type of grouping, there is a distinct level of concentration for each, for example, administration will have one value and agriculture another.

To some extent the percentage of women in an occupation depends on the extent of the employment of women in the labour force; the more women there are in employment, the more there are likely to be in any particular occupation. To take account of this, the female percentage in an occupation is sometimes divided by the female percentage of the employed labour force. This ratio is often referred to as the over-representation or under-representation of women in an occupation (according to whether its value is greater or less than one). This is not really a different conception of concentration, but rather a modification derived from the original measure.

Another way of examining concentration, which provides a useful overview, is to look at the distribution of workers across occupations with different degrees of female (or male) concentration. All of the occupations are grouped by their percentage female, then the number of workers can be plotted for levels of concentration (say at 10% intervals), showing the extent to which workers are employed in predominantly male, female, or mixed occupations.

(iii) Technical Points about the Data and Standardising MM

In order to estimate 'expected' values of the segregation measure, MM, for different numbers of occupations we needed to estimate the parameters of a non-linear regression line (see text for details of the equation). For the data, we combined MM values from several data sets. We used the ILO data set (excluding Romania, Senegal, and China which did not produce reliable measurements of MM) with countries ranging in number of occupations from 24 to 1050. Data presented by Rubery and Fagan (1995, p. 220) on nine countries (excluding only Luxembourg and Spain from their data set since we had equivalent ILO measures for these) were added. These measures were based on the ISCO (68) 2 digit data, approximately 80 occupations per country (varying a little as different categories were missing in different countries). The measures were less precise than our own, as recorded to only 2 decimal places, but this did not make much difference. As expected the segregation estimates were lower than figures we had calculated from more detailed occupational classifications for countries which our data sets had in common (e.g. the Rubery and Fagan measure for the UK is .56 compared to our 526 occupations measure of .635). To these measures were added MM values calculated from Labour Force Survey (LFS) data for the United Kingdom, for the odd years inclusive from 1981 through to 1991, and the first quarter data for the years from 1992 through to 1996. Calculations from the LFS data prior to 1991 were carried out for both the three digit classification (approximately 530 occupations) and the two digit level (approximately 161 occupations). Due to change in the LFS coding scheme, measures from 1991 onward were calculated at the three digit level, each being based on 371 occupations. The three digit level actually lists 374 occupational groupings, but we chose to omit vague 'miscellaneous' categories, and so 3 of the 374 categories in post- 1990 LFS data were excluded. Slight variation in the number of occupations included in the calculations from the LFS data for the 1980s arose because, for some years, particular occupational groupings had no respondents. Finally, the data for the United Kingdom 1991 Census were added. These data provided measures of MM for occupations classified to four levels: major (9 occupations), sub-major (22 occupations), minor or two digit level (77 occupations) and unit group or three digit level (371 occupations).

In estimating the regression equation we were concerned to take account of differences due to changes over time as well as differences across countries. Our historical data, taken mainly from the UK, might suggest a national bias. However, the method is not really affected in this way, and so far as we could judge there was no problem.

Using these data we derived the most appropriate equation to express the relation between the number of occupations and the expected values of MM. The equation should have the following constraints: MM = 0 for no segregation, which necessarily occurs when the number of occupations = 1; MM = 1 for complete segregation, which necessarily occurs when the number of occupations equals the number of people in the employed population; and MM increases as the number occupations increases. The second criterion could not be met perfectly by our estimating equation, because it is dependent on the actual number of workers in each labour force. We therefore chose an asymptotic approach to a value of 1 as the number of occupations approaches infinity, while the estimated value is very close to unity for the number of workers in all of the countries in our data. Since the number of occupations in even the most detailed occupational scheme is far short of the number of workers, for example, Bahrain in the ILO data set (1050 occupations), the function serves precisely as intended.

Once we had the appropriate estimating equation we needed to choose a particular number of occupations on which to standardise. We chose 200 occupations as this was towards the middle of the range of sizes for the national data sets, and because it represents a level where estimates are reliable. For low numbers of occupations, any increase in numbers has a large effect, but at 200 occupations this is no longer so; therefore any small errors in the equation can have little effect.

Beyond these basic constraints, the criteria on which the parameter values for the weighting formula were chosen are as follows:

1) A high and significant correlation fitting the data to our equation. We used the non-linear regression function in the SPSS for Windows software programme, and achieved a value of R² of .6088.

2) It was evident that the two outliers on the upper tail of the ILO data, Finland and Bahrain, had high levels of segregation even when the number of occupations was standardised. We were concerned that the standardization equation should not excessively deflate the coefficients estimated for 200 occupations in the countries. This meant we were not looking for the best fitting regression curve; we were balancing the smallest reduction in the correlation coefficient against the least reduction in the standardised values for these countries, given that the other criteria for the choice of the formula parameters were met.

3) As a check we estimated equations with and without the value of 1 for a huge number of occupations, and with the exclusion of the two outliers with large numbers of occupations. With the number of occupations tending to infinity, the value of R² increased but the equation was unaffected. Dropping the outliers produced a percentage change of about 1 in the value of R² for the unchanged equation.

4) Since the British Census provided good quality data for 4 different sized occupational groupings, we looked for consistency across their respective standardized measures of MM. Because the estimates based on larger numbers of occupations are less subject to error, we gave particular attention to the two values based on 371 and 77 occupations; the estimates for the grouping with 9 occupations were somewhat out of line with the others, confirming our belief that this grouping was comprised of too few occupations to yield a reliable measure, and so this did not affect our solution. We also had French data with two levels of occupational classification, which provided a further check on our estimates. The way occupations are grouped is bound to have some effect, so that it is not possible to get identical results when we standardise for different groupings. However, it emerged that the estimates were pretty consistent.

If data had been available it might have been worth checking on more countries. Nevertheless, the regression curve is very stable, in the sense that modest variations in the parameters have very little effect, so it is most unlikely that further tests would have had any significant consequence.

Notes

¹ For example, see the Draft Platform for Action, United Nations, Fourth World Conference on Women, Beijing, China, 4-15 September 1995.

² The terms 'segregation' and 'concentration' are used to differentiate different types of data patterns. Some analysts refer to all of these in an undifferentiated way as 'segregation'; however, we find this confusing. We use the term 'segregation' when we are referring to the tendency for men and women to be employed in different occupations across an entire spectrum of occupations. 'Concentration' refers to the representation of one sex within one or a group of occupations. For a further discussion and definitions, see Appendix 'Key Concepts'.

³ The book by Anker and Hein (1986) is an important contribution in this area, including case studies and macro level data for Cyprus, the city of Lucknow in India, the city of Colombo in Sri Lanka, the city of Accra-Tema in Ghana, Mauritius, and the city of Lima, Peru. Their focus, however, is primarily on urban employment.

⁴ This assessment of the literature is based on extensive searches in databases such as SOCIOFILE, and BIDS. Material written in languages not referenced by these sources will not be represented.

⁵ Some of these reasons include lack of importance placed on informal sector activity by governments, gender bias in the construction of official categories, a narrow focus and definition of economic activity as geared solely to the production of goods and services as commodities rather than as contributions to livelihood, the unwillingness of respondents to make unofficial economic activity visible to government officials. There have been some interesting efforts, however, to improve the sensitivity of official statistics with respect to the informal sector (e.g. Anker and Anker, 1989; Anker and Dixon-Mueller, 1988; Anker et al, 1987). While it has become a convention in feminist circles to work towards making women's work more visible, especially to government and development agencies, it may be the case that this would have a disempowering effect as it may simply increase the opportunities for state control which may not always be positive for the women concerned (See Ferguson, 1994, for a thought-provoking discussion of the effects of extending government power, although he does not discuss gender issues at great length).

⁶ As already noted some countries have even fewer categories (e.g. Mexico with 17), but these are regarded as having too few categories for useful analysis.

⁷ It is difficult to predict whether an unemployed worker will find employment within the same occupation or not.

⁸ See Appendix, 'Segregation', for a discussion of choice of measure.

⁹ Thus, if MM_ni is the observed value of MM in country i where the data set has n occupations and MM_nE is the expected value for n occupations, we estimate MM_200i = MM_200E x MM_ni / MM_nE.

¹⁰ We should note that this meets three basic criteria: MM_E = 0 when n = 1; MM_E increases as n increases; and MM_E 1 as n . The third criterion here is not precisely what is required, but the difference is negligible, as explained in the Appendix.

¹¹ Bahrain's economy is unusual because of its heavy reliance on migrant workers. Only 42% of its labour force is composed of citizens of Bahrain (Bahrain Human Rights Organisation (1995) www.iae.dtu/u/d946801/bhro2.htm).

¹² We use the concepts of 'vertical' and 'horizontal' in their usual mathematical sense, rather than the unusual sense sometimes employed in segregation research. Most confusing in other segregation research is the use of the term 'horizontal' segregation to apply to what is actually the resultant of mathematically vertical and horizontal components (e.g. Hakim 1979, Moore 1985, Rubery and Fagan 1995).

Acknowledgements

We should like to thank the following for their support: the ILO, particularly Eivind Hoffman and Adriana Mata-Greenwood; the United Nations Statistical Division, particularly Francesca Perucci; the British Economic and Social Research Council (grant number R000236844) and the Canadian Social Sciences and Humanities Research Council.

References

ANKER, R. and ANKER, M. (1989) 'Measuring the Female Labour Force in Egypt', International Labour Review, Vol. 128, No. 4, pp. 511-20.

ANKER, R. and DIXON-MUELLER, R. (1988) Assessing Women's Contribution to Economic Development. Geneva: International Labour Office.

ANKER, R. and HEIN, C. (1986) 'Sex inequalities in third world employment: Statistical evidence' in R. Anker and C. Hein (editors) Sex Inequalities in Urban Employment in the Third World. London: Macmillan.

ANKER, R., KHAN, M.E. and GUPTA, R.B. (1987) 'Biases in Measuring the Labour Force Results of a Methods Test Survey in Uttar Pradesh, India', International Labour Review, No. 2.

BLACKBURN, R.M., BROOKS, B., and JARMAN, J. (1999) Gender Inequality in the Labour Market: The Vertical Dimension of Occupational Segregation. Cambridge Studies in Social Research No. 3, Cambridge: SRG Publications.

BLACKBURN, R.M., JARMAN, J., and BROOKS, B. (1999) The Relation Between Gender Inequality and Occupational Segregation in 32 Countries. Cambridge Studies in Social Research No. 2, Cambridge: SRG Publications. BLACKBURN, R.M. and JARMAN, J. (1997) 'Occupational Gender Segregation', Social Research Update, No. 16, Spring, <http://www.soc.surrey.ac.uk/sru/SRU16/SRU16.html>.

BLACKBURN, R.M., JARMAN, J. and SILTANEN, J. (1993) 'The Analysis of Occupational Gender Segregation Over Time and Place: Considerations of Measurement and Some New Evidence', Work, Employment and Society, Vol. 7, No. 3, pp. 335-362.

BLACKBURN, R.M. and MARSH, C. (1991) 'Education and Social Class: Revisiting the 1944 Education Act with Fixed Marginals.' British Journal of Sociology, Vol. 42, No. 4, pp. 507- 536.

BLAU, F.D. and FERBER, M.A. (1992) The Economics of Women, Men, and Work. New Jersey: Prentice Hall.

BRADLEY, H. (1989) Men's Work, Women's Work. Cambridge: Polity Press.

CHARLES, M. (1992) 'Accounting for cross-national variation in occupational sex segregation', American Sociological Review, Vol. 57, No. 4, pp. 483-502.

CHARLES, M. and BUCHMANN, M. (1994) 'Assessing Micro-Level Explanations of Occupational Sex Segregation: Human Capital Development and Labour Market Opportunities in Switzerland.' Swiss Journal of Sociology, vol. 20, no. 3.

CONNELL, B. (1987) Gender and Power. Cambridge: Polity Press.

CROMPTON, R. and SANDERSON, K. (1990) Gendered jobs and social change. London: Unwin Hyman.

ENGLAND, P. (1981) 'Assessing trends in occupational sex segregation, 1900-76.' in I. Berg (editor) Sociological Perspectives on Labour Markets. New York: Academic Press.

FERGUSON, J. (1994) The Anti-Politics Machine, 'Development', Depoliticization, and Bureaucratic Power in Lesotho. Minneapolis: University of Minnesota Press.

HAKIM, C. (1979) Occupational Segregation: A Comparative Study of the Degree and Pattern of the Differentiation Between Men and Women's Work in Britain, the United States and Other Countries, Research Paper No. 9. London: Department of Employment.

HAKIM, C. (1992) 'Explaining Trends in Occupational Segregation: The Measurement, Causes and Consequences of the Sexual Division of Labour', European Sociological Review, Vol. 8, No. 2, pp. 127-152.

HAKIM, C. (1993a) 'Segregated and integrated occupations: a new framework for analyzing social change', European Sociological Review, Vol. 9, No. 3, pp. 289-314.

HAKIM, C. (1993b). 'Refocusing Research on Occupational Segregation: Reply to Watts', European Sociological Review, Vol. 9, No. 3, pp. 321-324.

HAKIM, C. (1996) Female Heterogeneity and the Polarisation of Women's Employment. London: Athlone.

HUSSMANNS, R., MEHRAN, F. and VERMA, V. (1990) Surveys of Economically Active Population, Employment, Unemployment and Underdevelopment. Geneva: International Labour Office.

JACOBS, J. and LIM, S. (1992) 'Trends in occupational and industrial sex segregation in 56 countries: 1960-80', Work and Occupations, Vol. 19, No. 4, pp. 450-486.

MOORE, G. (1985) 'Horizontal and Vertical: The Dimensions of Occupational Segregation by Gender in Canada', The CRIAW Papers, No. 12: Canadian Research Institute for the Advancement of Women.

RESKIN, B. (1993) 'Sex Segregation in the Workplace', Annual Review of Sociology, Vol. 19, pp. 241-270.

RESKIN, B. and ROOS, P. (1990) Job Queues, Gender Queues - Explaining Women's Inroads into Male Occupations. Philadelphia: Temple University Press.

ROOS, P. (1985) Gender and Work: A Comparative Analysis of Industrial Societies. New York: SUNY Press.

RUBERY, J. and FAGAN, C. (1993) Occupational Segregation of Women and Men in the European Community. Social Europe Supplement 3/93, Luxembourg: CEC.

RUBERY, J. and FAGAN, C. (1995) 'Gender Segregation in Societal Context', Work, Employment and Society, Vol. 9, No. 2, pp. 213-240.

SILTANEN, J. (1990) 'Social Change and the Measurement of Occupational Segregation by Sex: An Assessment of the Sex Ratio Index', Work, Employment and Society, Vol. 4, No. 1, pp. 1-29.

SILTANEN, J., JARMAN, J. and BLACKBURN, R.M. (1995) Gender Inequalities in the Labour Market. Geneva: International Labour Office.

WALBY, S. (1988) Gender Segregation at Work. Milton Keynes: Open University Press.

Copyright Sociological Research Online, 1999